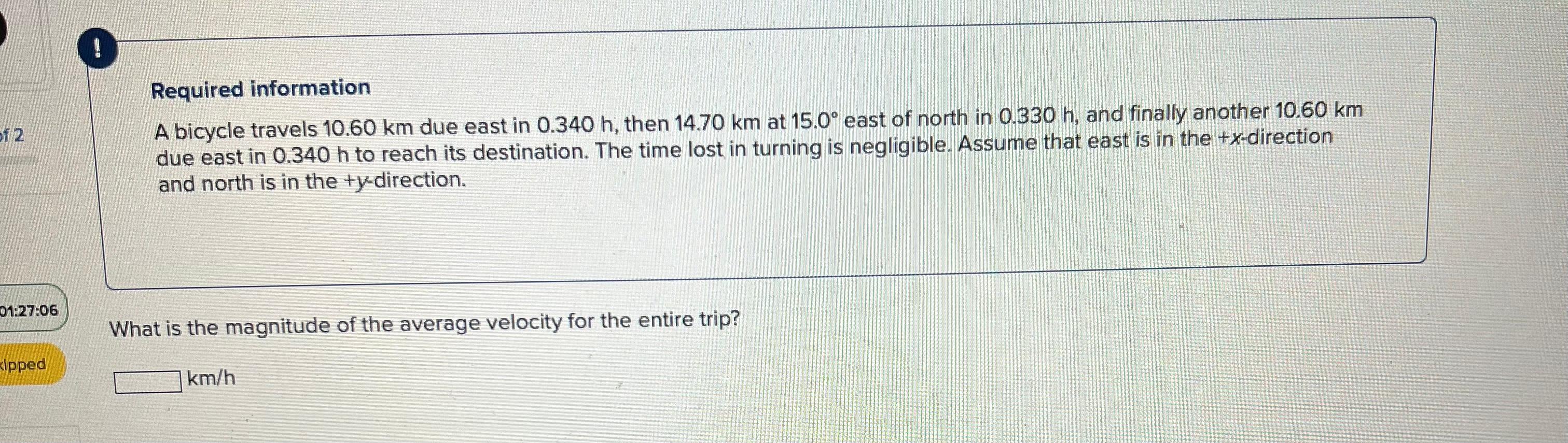
A biker rides 700m north 300m east – A biker rides 700m north, 300m east – a seemingly simple journey that reveals intriguing concepts in physics. This scenario allows us to explore the difference between distance and displacement, two fundamental concepts in understanding motion. By analyzing the biker’s path, we can delve into how these concepts are applied in real-world situations, such as navigation and mapping.
Imagine a biker embarking on a journey, first heading north for 700 meters and then turning east for 300 meters. This straightforward path offers a perfect illustration of how distance and displacement differ. Distance refers to the total length traveled, while displacement considers the straight-line distance between the starting and ending points. In our biker’s journey, the total distance is the sum of the north and east movements, while the displacement is the hypotenuse of a right triangle formed by the biker’s path.
Understanding the Movement
The biker’s journey can be visualized as a combination of two distinct movements: one northward and the other eastward. This approach allows us to analyze the biker’s path and determine the overall distance traveled and the final position relative to the starting point.
Visualizing the Path
The biker’s route can be represented as a right triangle, where the northward movement forms one leg, the eastward movement forms the other leg, and the hypotenuse represents the overall distance traveled.
The biker’s path is represented by a right triangle, with the northward movement as one leg, the eastward movement as the other leg, and the hypotenuse representing the overall distance traveled.
Calculating Distance
The biker’s journey can be visualized as a right triangle, with the northward movement representing one leg and the eastward movement representing the other leg. To determine the total distance traveled, we need to calculate the hypotenuse of this triangle, which represents the direct path from the starting point to the ending point.
Using the Pythagorean Theorem
The Pythagorean theorem states that in a right triangle, the square of the hypotenuse (c) is equal to the sum of the squares of the other two sides (a and b):
c² = a² + b²
In this case, the northward movement (700m) is ‘a’, the eastward movement (300m) is ‘b’, and the total distance traveled (c) is the hypotenuse.Let’s plug the values into the formula:
c² = 700² + 300²
c² = 490,000 + 90,000
c² = 580,000
To find the value of c, we need to take the square root of both sides:
c = √580,000
c ≈ 761.58 m
Therefore, the total distance traveled by the biker is approximately 761.58 meters.
Displacement
Displacement is a fundamental concept in physics that describes the overall change in an object’s position. It’s a vector quantity, meaning it has both magnitude (size) and direction. Unlike distance, which measures the total path traveled, displacement focuses on the straight-line distance between the starting and ending points.
Calculating the Biker’s Displacement
The biker’s displacement can be visualized as a straight line drawn from the starting point to the ending point. This line represents the shortest distance between the two points, regardless of the actual path the biker took.To calculate the biker’s displacement, we can use the Pythagorean theorem, which states that in a right triangle, the square of the hypotenuse (the longest side) is equal to the sum of the squares of the other two sides.In this case, the biker’s northward journey represents one side of the right triangle, and the eastward journey represents the other side.
The displacement is the hypotenuse of this triangle.
Displacement = √(Northward Distance² + Eastward Distance²)
Displacement = √(700m² + 300m²)
Displacement = √(490000m² + 90000m²)
Displacement = √(580000m²)
Displacement ≈ 761.58m
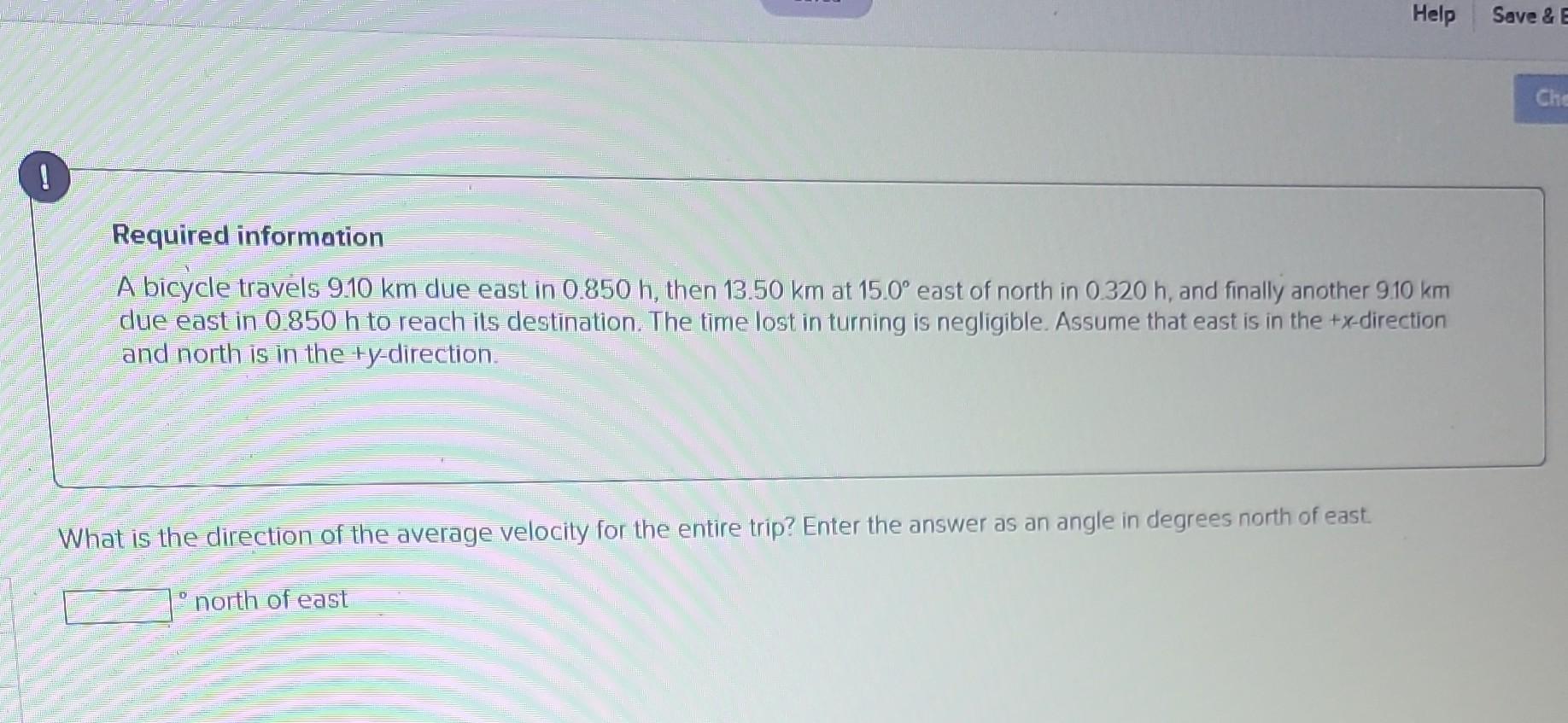
Direction of Displacement
The direction of the biker’s displacement is not simply north or east. Instead, it’s a combination of both directions, forming an angle with respect to the horizontal axis (east). This angle can be determined using trigonometry.
tan(θ) = (Northward Distance) / (Eastward Distance)
tan(θ) = 700m / 300m
θ = arctan(7/3)
θ ≈ 66.8°
Therefore, the biker’s displacement is approximately 761.58 meters at an angle of approximately 66.8° north of east. This means the biker ended up 761.58 meters away from the starting point in a direction that’s slightly more north than east.
Visual Representation
A visual representation of the biker’s journey helps us understand the path taken and the overall displacement.
Table Representation
A table can be used to summarize the biker’s movements:
| Direction | Distance (m) |
|---|---|
| North | 700 |
| East | 300 |
Diagram Representation
A scaled diagram can visually depict the biker’s journey.
Imagine a coordinate plane with the starting point at the origin (0, 0). The biker first travels 700 meters north, reaching a point (0, 700). Then, they travel 300 meters east, ending at the point (300, 700).
The diagram would show a right triangle with the following:* Hypotenuse: The biker’s overall displacement.
Vertical Leg
The distance traveled north (700 meters).
Horizontal Leg
The distance traveled east (300 meters).This diagram allows for a clear visual understanding of the biker’s path and the resulting displacement.
Real-World Application: A Biker Rides 700m North 300m East
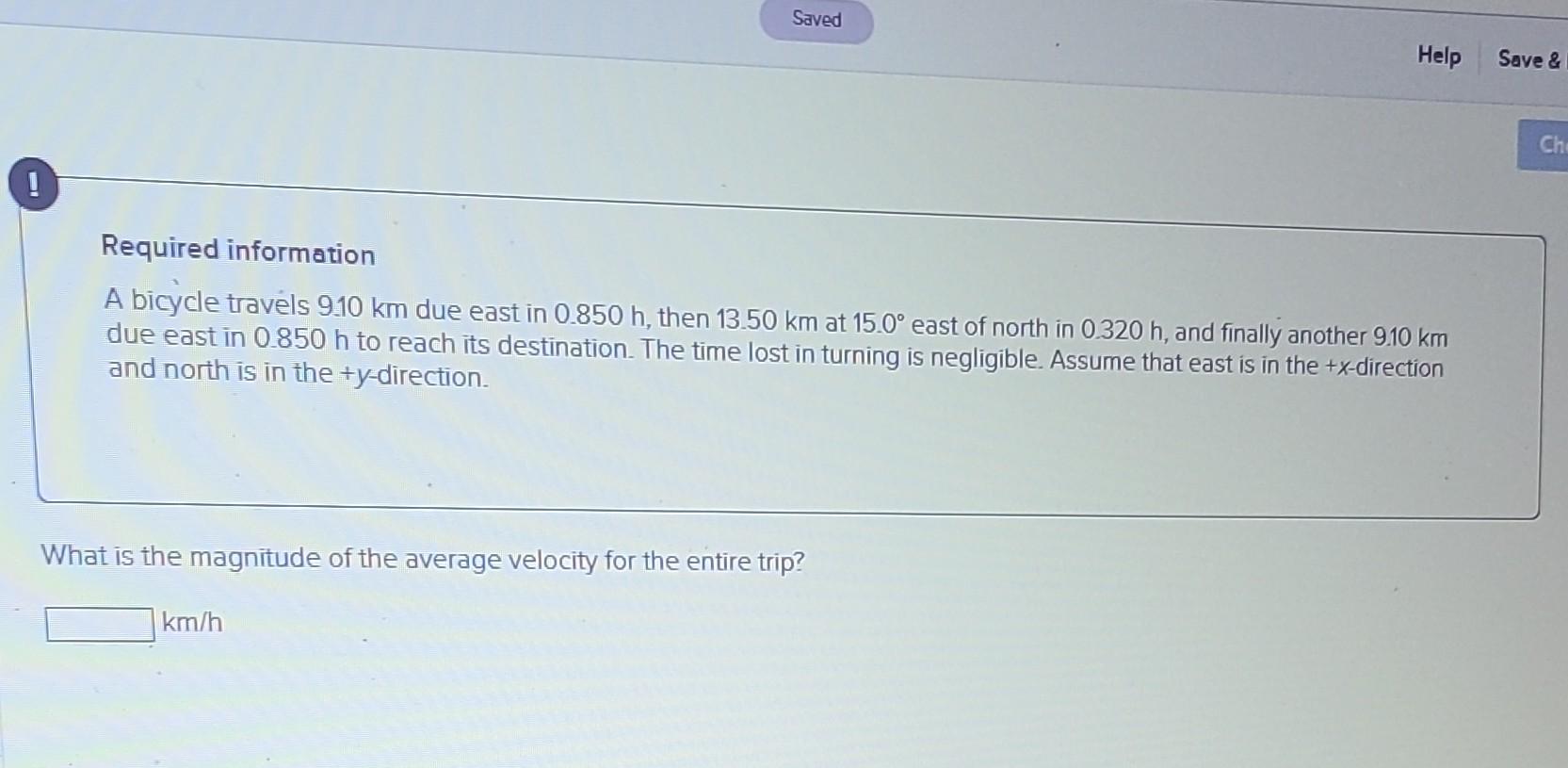
The scenario of a biker traveling 700 meters north and then 300 meters east can be applied to various real-world situations, especially in navigation and mapping. Understanding the concepts of distance and displacement is crucial in these contexts, as they help determine the biker’s actual path and the shortest route to their destination.
Navigation and Mapping, A biker rides 700m north 300m east
In navigation and mapping, understanding distance and displacement is crucial for accurate route planning and determining the shortest distance between two points. The biker’s journey demonstrates this:
- The biker’s total distance traveled is 1000 meters (700 meters north + 300 meters east).
- The biker’s displacement, however, is the straight-line distance between their starting and ending points. This can be calculated using the Pythagorean theorem:
Displacement = √(700² + 300²) ≈ 761.58 meters
This difference between distance and displacement is crucial for navigation systems, which use displacement to calculate the shortest route, while also considering factors like traffic and road conditions.
Importance of Distance and Displacement
Distance and displacement are fundamental concepts in physics and everyday life. Understanding these concepts is important for:
- Accurate route planning: Knowing the shortest distance between two points helps in efficient travel and resource allocation.
- Understanding motion: Displacement provides a clear representation of an object’s change in position, while distance accounts for the actual path traveled.
- Mapping and surveying: Accurate measurements of distance and displacement are crucial for creating maps and surveying land.
Relation to the Biker’s Journey
In the biker’s journey, the concepts of distance and displacement are clearly demonstrated. The biker’s total distance traveled is 1000 meters, while their displacement is approximately 761.58 meters. This difference highlights the importance of considering both concepts in real-world scenarios.
Understanding the difference between distance and displacement is crucial in various real-world applications. From navigating a city to planning a long road trip, these concepts help us make informed decisions about our journeys. By applying the principles of distance and displacement, we can efficiently navigate our surroundings, optimize our routes, and make the most of our travels. The biker’s journey, though seemingly simple, provides a valuable lesson in understanding these fundamental concepts and their practical implications.
General Inquiries
How does the Pythagorean theorem apply to this scenario?
The Pythagorean theorem helps us calculate the displacement, which is the hypotenuse of the right triangle formed by the biker’s north and east movements. The theorem states that the square of the hypotenuse is equal to the sum of the squares of the other two sides. In this case, the displacement is the square root of (700^2 + 300^2), which gives us the straight-line distance between the starting and ending points.
What is the biker’s displacement?
The biker’s displacement is the straight-line distance between the starting and ending points. To calculate it, we use the Pythagorean theorem: displacement = √(700^2 + 300^2) = √(490000 + 90000) = √580000 ≈ 761.58 meters.
What is the direction of the biker’s displacement?
The direction of the biker’s displacement is the angle relative to the horizontal axis (east). We can calculate this using trigonometry: tan(theta) = opposite/adjacent = 700/300, so theta = arctan(700/300) ≈ 66.8 degrees north of east.