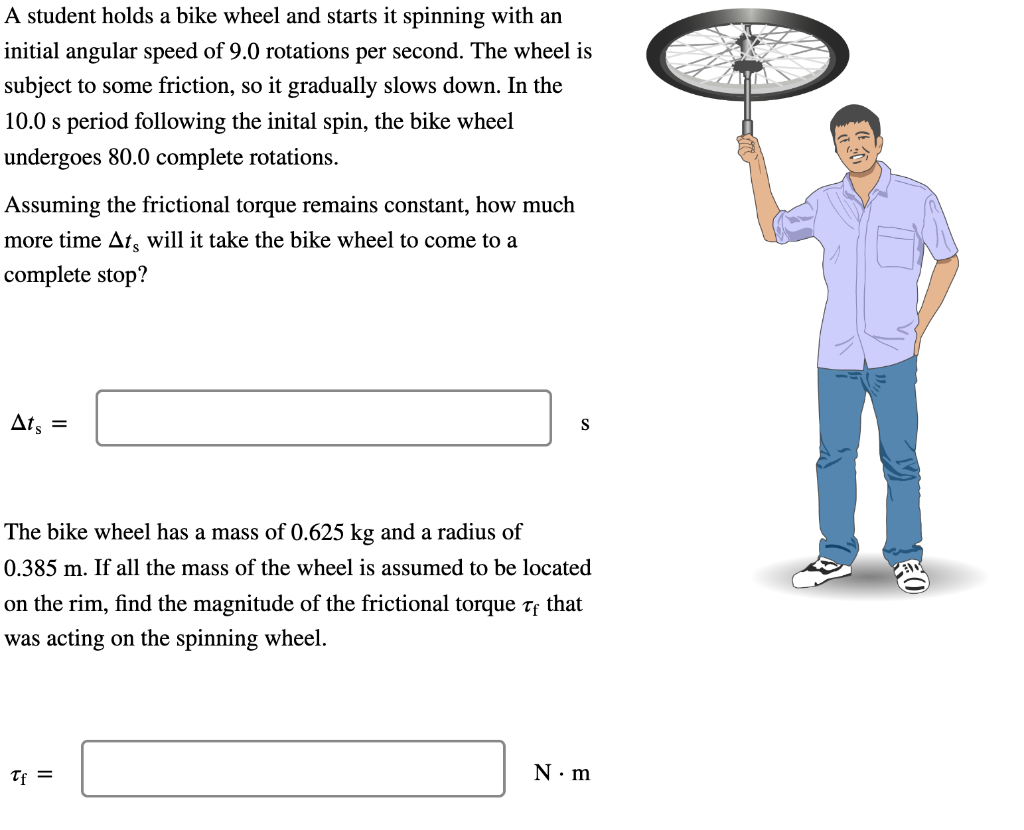
A student holds a bike wheel and starts it spinning, setting the stage for an exploration of the captivating interplay between physics and everyday experience. This simple act, seemingly mundane, becomes a gateway to understanding fundamental concepts like angular velocity, torque, and the conservation of angular momentum. As the wheel spins, a mesmerizing visual spectacle unfolds, blurring the spokes and creating an illusion of continuous motion.
The student, now a witness to the magic of physics, is transported into a world where the ordinary becomes extraordinary.
This exploration delves into the intricate mechanics of spinning, revealing the hidden forces that govern the wheel’s motion. We’ll delve into the fascinating relationship between angular velocity, torque, and inertia, unraveling the secrets behind the wheel’s graceful spin. The concept of conservation of angular momentum, a cornerstone of physics, will be unveiled, explaining why the wheel continues to spin with seemingly endless energy.
The Physics of Spinning
When a student spins a bike wheel, they are engaging with fundamental principles of physics, particularly those related to rotational motion. The spinning wheel exhibits several forces, and the interplay between them governs its behavior.
Angular Velocity, Torque, and Inertia, A student holds a bike wheel and starts it spinning
The rate at which the wheel spins is known as its angular velocity. It is measured in radians per second (rad/s). The force responsible for initiating and changing the angular velocity is called torque. Torque is a rotational force, and it depends on the magnitude of the applied force and the distance from the axis of rotation.
The bike wheel’s resistance to changes in its rotational motion is known as its moment of inertia. The moment of inertia is a measure of how difficult it is to start or stop the wheel’s rotation.
The relationship between angular velocity (ω), torque (τ), and moment of inertia (I) is given by the following equation:τ = Iαwhere α is the angular acceleration.
Conservation of Angular Momentum
The angular momentum of a spinning object is a measure of its rotational inertia. It is calculated by multiplying the object’s moment of inertia by its angular velocity. In the absence of external torques, the angular momentum of a system remains constant. This principle, known as the conservation of angular momentum, explains why the spinning bike wheel continues to rotate at a constant speed once it is set in motion.
The conservation of angular momentum is represented by the following equation:L = Iω = constantwhere L is the angular momentum.
The Visual Experience: A Student Holds A Bike Wheel And Starts It Spinning
The spinning bike wheel presents a captivating visual spectacle, a mesmerizing interplay of motion, blurring, and changing patterns. The spokes, normally distinct and static, transform into a blur of colors and shapes as the wheel gains speed. This visual phenomenon, a result of the wheel’s rapid rotation and the limitations of our visual perception, provides a fascinating glimpse into the workings of our eyes and brains.
Persistence of Vision
The apparent blurring of the spokes is a consequence of a fascinating aspect of human vision known as persistence of vision. Our eyes don’t perceive the world as a series of static snapshots but rather as a continuous flow of images. This phenomenon, where the brain retains an image for a brief period after it is no longer visible, is responsible for the smooth perception of motion in movies and animations.
- When a spinning wheel is viewed, the spokes appear blurred because our eyes are unable to track their rapid movement. Instead, our brains blend the images of the spokes as they pass by, creating the illusion of a continuous blur.
- The speed at which the wheel spins influences the extent of blurring. A slower spinning wheel will show less blurring, allowing us to discern the individual spokes more easily. As the speed increases, the blurring becomes more pronounced, making it difficult to distinguish the spokes.
Psychological Effects of Spinning Objects
The visual experience of a spinning object, like a bike wheel, can have a profound impact on our perception and evoke a range of psychological responses.
- The constant movement of the spinning wheel can induce a sense of motion, even if we are stationary. This can be a powerful effect, drawing our attention to the object and creating a sense of dynamism.
- The hypnotic effect of a spinning object can be attributed to the repetitive nature of the movement. The continuous rotation can be soothing and calming, helping to focus our attention and block out distractions.
- The visual experience can also evoke a sense of wonder and curiosity. The transformation of the spokes from distinct lines to a blur of color and shape can be captivating, prompting us to explore the science behind this phenomenon.
The Practical Applications
The spinning of a bike wheel is not merely a visually captivating phenomenon; it is the very foundation of a bicycle’s functionality. The spinning wheel, with its inherent properties of inertia and angular momentum, plays a crucial role in enabling a bicycle to move and maintain its balance.
The Role of Spinning in Bicycle Motion
The spinning of a bike wheel directly contributes to the bicycle’s motion. As the wheel rotates, it exerts a force on the ground, propelling the bicycle forward. This force is generated due to the friction between the tire and the ground. The faster the wheel spins, the greater the force exerted, resulting in increased acceleration.
Gyroscopic Stability
Gyroscopic stability is a fundamental principle that explains how a bicycle maintains its balance. A spinning object, like a bike wheel, possesses angular momentum, which is a measure of its tendency to continue rotating about its axis. This property allows the wheel to resist changes in its orientation, acting as a stabilizing force. When a bicycle leans, the spinning wheel’s angular momentum resists the tilting motion, causing the bicycle to right itself.
This effect is particularly pronounced at higher speeds, as the angular momentum of the wheel increases with its rotational velocity.
Examples of Spinning Objects in Various Fields
Spinning objects find widespread applications in various fields, showcasing the practical significance of this fundamental principle.
- Gyroscopes in Navigation Systems: Gyroscopes are devices that utilize the principle of angular momentum to maintain a fixed orientation in space. They are crucial components in navigation systems, ensuring accurate direction and position information. Gyroscopes are used in aircraft, ships, and satellites to provide precise orientation data, enabling them to navigate effectively.
- Spinning Turbines in Power Generation: Spinning turbines are essential for generating electricity. In power plants, turbines are driven by the flow of steam, water, or gas, converting their kinetic energy into mechanical energy. This mechanical energy is then used to rotate a generator, producing electricity. The spinning motion of the turbines is a fundamental aspect of power generation.
- Centrifuges in Laboratories: Centrifuges are devices that use spinning motion to separate components of a mixture based on their density. They are widely used in laboratories for various purposes, including blood analysis, DNA extraction, and purification of materials. The spinning motion creates a centrifugal force that pushes denser components to the outside of the centrifuge tube, enabling separation.
The Creative Exploration
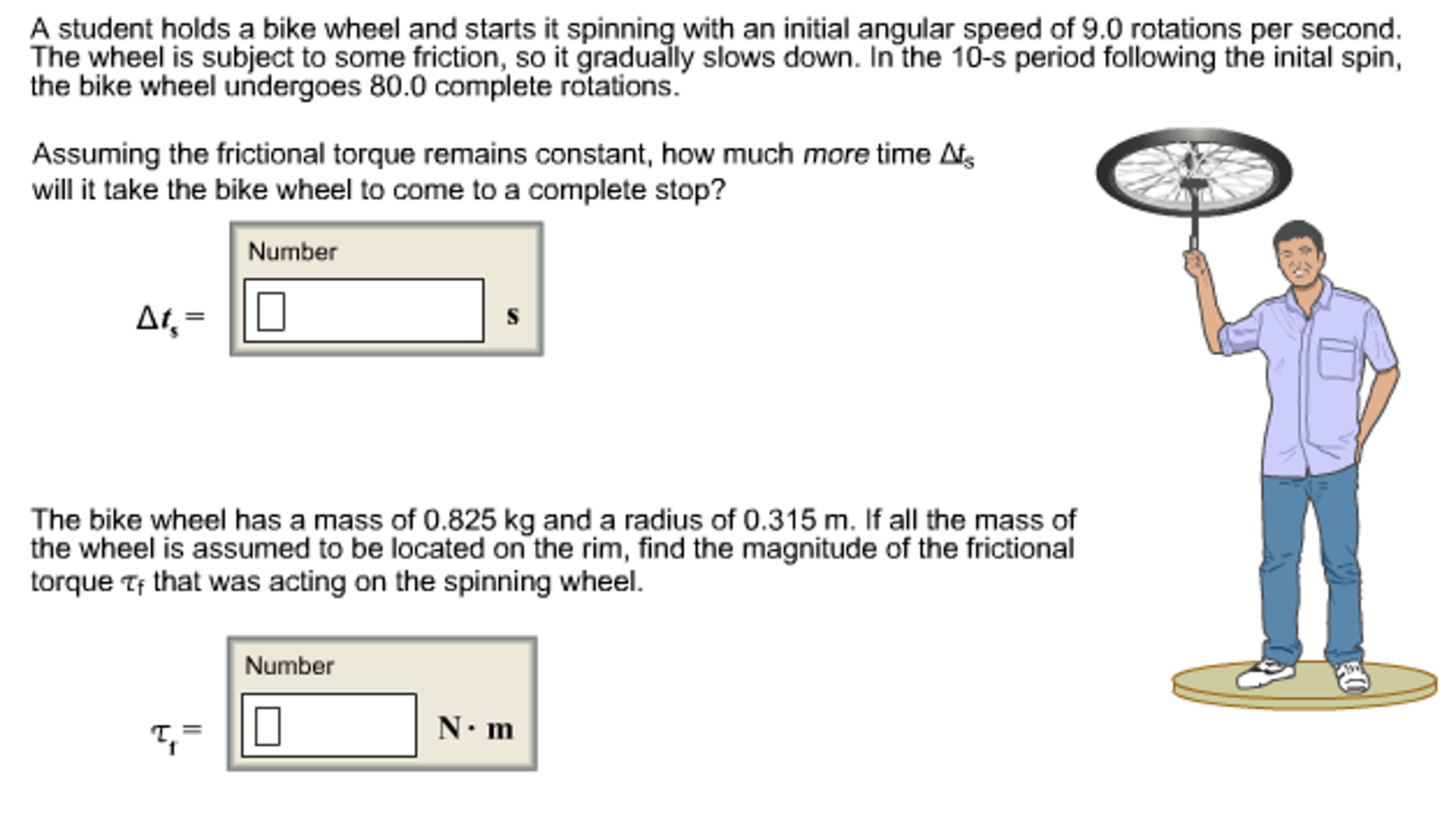
The spinning bike wheel, with its captivating motion and inherent energy, offers a rich canvas for creative exploration. Its dynamic nature inspires visual representations, evocative narratives, and artistic expressions that capture its essence.
Visual Representations
The visual representation of a spinning bike wheel can be achieved through various means, each emphasizing different aspects of its motion and energy.
- Circular Motion: A series of concentric circles, progressively smaller towards the center, can depict the wheel’s rotation. The circles can be filled with different colors or shades to represent the changing speed of the wheel.
- Radial Lines: Lines radiating from the center of the wheel, resembling spokes, can be used to visualize the wheel’s rotation. The lines can be curved or straight, depending on the desired effect.
- Blur Effect: The blurring of the wheel’s spokes as it spins can be captured by using a series of overlapping images, each slightly offset from the previous one. This creates a sense of motion and energy.
- Color Gradient: A gradient of colors can be used to depict the changing speed of the wheel. For example, a blue to red gradient can represent the wheel slowing down, while a red to blue gradient can represent the wheel speeding up.
Narrative Exploration
The image of a spinning bike wheel can serve as a powerful metaphor or symbol in narratives, representing concepts such as:
- Persistence: The relentless spinning of the wheel can symbolize the importance of perseverance and determination in achieving goals.
- Change: The continuous rotation of the wheel can represent the ever-changing nature of life and the constant flow of time.
- Progress: The forward motion of the spinning wheel can symbolize progress and advancement towards a desired destination.
- Energy: The kinetic energy of the spinning wheel can represent the energy and vitality that drive us forward.
Artistic Inspiration
The visual and kinetic qualities of a spinning bike wheel can inspire a wide range of artistic creations.
- Kinetic Sculpture: A sculpture that incorporates a spinning bike wheel as a central element, with other moving parts, can create a dynamic and engaging piece of art.
- Light Installation: A light installation featuring a spinning bike wheel, with LEDs attached to its spokes, can create a mesmerizing display of light and motion.
- Abstract Painting: An abstract painting that captures the blurred and fragmented imagery of a spinning bike wheel can evoke a sense of movement and energy.
- Digital Animation: A digital animation that simulates the spinning of a bike wheel can explore various visual effects and creative possibilities.
From the mesmerizing visual effects of the spinning wheel to its practical applications in the world around us, the simple act of a student spinning a bike wheel opens a window into a world of scientific wonder. The blurring spokes, the hypnotic effect of motion, and the underlying principles of physics all converge to create a captivating experience that transcends the ordinary.
The spinning wheel, a seemingly mundane object, becomes a catalyst for understanding the intricate workings of the universe, reminding us that even the simplest of actions can hold profound scientific significance.
FAQ Compilation
Why does the bike wheel spin for so long?
The bike wheel spins for a long time due to the principle of conservation of angular momentum. This principle states that the total angular momentum of a system remains constant in the absence of external torques. In this case, the wheel’s initial angular momentum is maintained as it spins, allowing it to continue rotating for an extended period.
What happens to the spokes when the wheel spins?
When the wheel spins, the spokes appear blurred due to the persistence of vision. Our eyes retain an image for a fraction of a second, and as the spokes move quickly, they create a continuous blur, giving the illusion of a solid disk. This effect is similar to how a moving fan blade appears as a solid disk when rotating rapidly.
How does the spinning bike wheel relate to the function of a bicycle wheel in motion?
The spinning bike wheel is essential for the function of a bicycle. It provides stability through gyroscopic forces, which help the bicycle stay upright. When the wheel spins, it resists changes in its orientation, creating a stabilizing effect that allows the cyclist to maintain balance.